Which of the following definite integrals could be used to calculate the total area bounded by the graph of y = sin(x), the xaxis, x = 0, and x = π a) ∫ from π to 0 sin(x)dx b) ∫ from π to 0 sin(x)dx c) 2∫ from π to 0 You can view more similar questions or ask a new questionIf Y = Sin1 X Cos1x Find (Dy)/(Dx) CBSE CBSE (Commerce) Class 12 Question Papers 1786 Textbook Solutions Important Solutions 3417 Question Bank Solutions Concept Notes & Videos 532 Time Tables 18 Syllabus Advertisement Remove all adsFind dy/dx y=x \sin x^{2} 🚨 Hurry, space in our FREE summer bootcamps is running out 🚨

Question 13 On Given Cos X Given Cos X Y Sin X Chegg Com
30.if y=(sin x)^(x)+x^(cos x) find (dy)/(dx)
30.if y=(sin x)^(x)+x^(cos x) find (dy)/(dx)-In the diagram above , XZ is the diameter of the circle XZW, with center O and r PQR is the diagram of a semicircle RSP with centre at Q and radius of length 35 If y = 3 cos 4x, dy/dx equals In the figure, XYZ is a triangle with XY = 5cm, XZ = 2cm and XZ is produced to E If x varies directly as y 3 and x = 2 when y = 1, find x when yRead it Question Find dy/dx by implicit differentiation x sin(y) y sin(x) = 2 x cos(y) sin(x) y' x sin(y) y cos(x) Need Help?




Find Dy Dx When If Y Cos X Sinx Cos X Sinx
solve (cosx tany cos (xy))dx (sin x sec 2 ycos (xy))dy=0 differentialequation derivatives asked in CALCULUS by linda ScholarCompute answers using Wolfram's breakthrough technology & knowledgebase, relied on by millions of students & professionals For math, science, nutrition, historyCalculus Find dy/dx y=sin (cos (x)) y = sin(cos (x)) y = sin ( cos ( x)) Differentiate both sides of the equation d dx (y) = d dx (sin(cos(x))) d d x ( y) = d d x ( sin ( cos ( x))) The derivative of y y with respect to x x is y' y ′ y' y ′ Differentiate the right side of the equation Tap for more steps
If sin x (dy/dx)y cos x=x sinx, then (y1) sin x= (A) cx sinx (B) cx cosx cx cosx (D) cx sinx Check Answer and Solution for above question f Answered Solved Solve differential equation sin(x) dy/dx(cos(x))y=0, y((7pi)/6)=2Y = sin x cos x 5 dy/dx = cos x sin x d2y/dx^2 = sin x cos x Further, the maximum occurs at cos x sin x or cos x = sin x or x = 45⁰ The maximum value of y = sin 45 cos 45 5 =
Solve (cosxcosycotx)dxsinxsinydy=0 A sin x cos y = ln (c cos x) B sin x cos y = ln (c sin x) C sin x cos y = ln (c sin x) D sin x cos y = ln (c cos x) Problem Answer The solution is sin x cos y = ln (c sin x) View Solution Latest Problem Solving in Differential Equations y=tan^1(sin(x)cos(x)) dy/dx=d/dx(tan^1(sin(x)cos(x))) = 1/1(sinxcosx)^2 x d/dx(sinxcosx) = 1/1(sinxcosx)^2 x cosxsinx = (cosxsinx)/(sinxcosx)^2Y = (sin x) x ∴ log y = log (sin x) x ∴ log y = x log sin x ∴ `1/y dy/dx = x 1/sin x cos x log sin x1` ∴ `dy/dx = y x cot x log sin x `




If Y Xsinx Cosx Xcosx Sinx Then Find Dy Dx Youtube
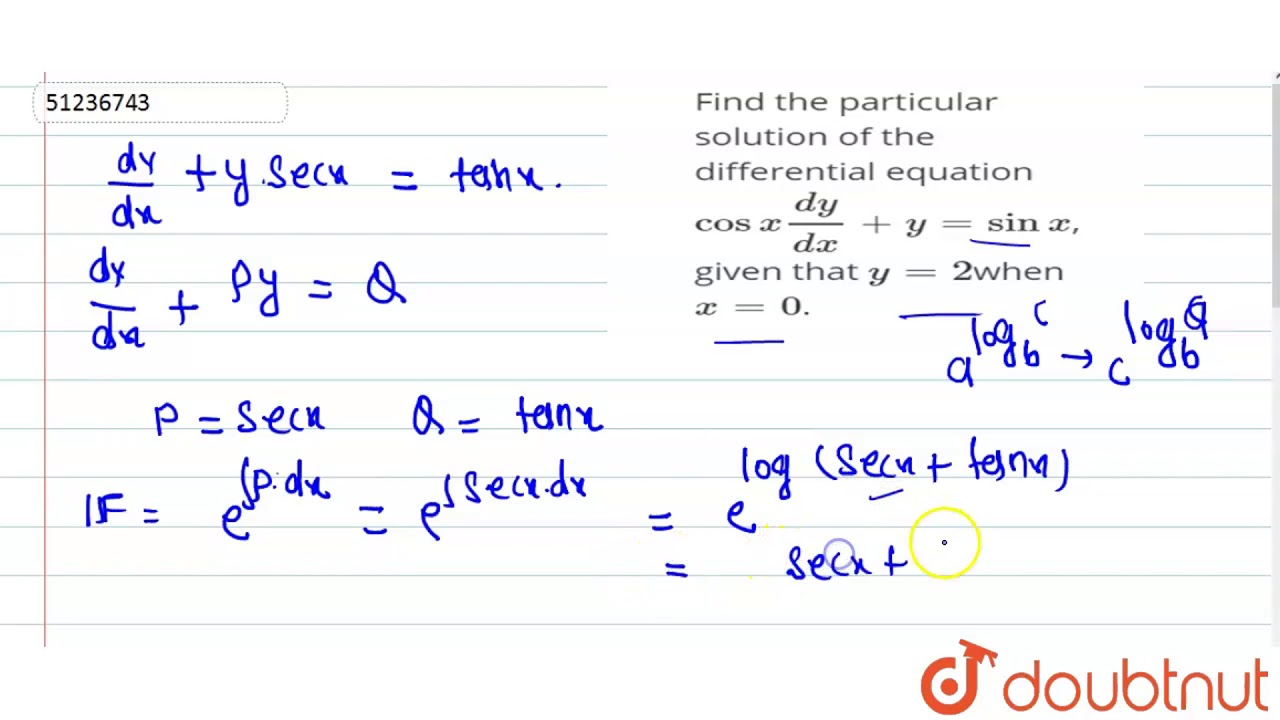



Find The Particular Solution Of The Differential Equation Cos X Dy Dx Y Sin X Youtube
Y = d d x ( sin x × log e x) In this differentiation problem, the variable y represents a function in x Hence, it can be differentiated with respect to x and do not think that y is a constant Therefore, the function y can be differentiated by the derivative rule of logarithms 1 y × d y d x = d d x ( sinImage transcriptions ( 2 x y cos x 2 _ 2 x y 1 ) dx ( sin x 2 x 2 ) dy = 0 first order linear ordinary differential Equates A first order linear ODE has the form y' ( 4) p(xjy = 9 ( x ) let y be the dependent variable Divide by dx If zug coslay any ( sin 123 29 dy da sub stitude with yo rewrite this into form of linear ODE y 2 2 ( cos (29 1 ) y 1 = 212 sin (my sin 107Differentiating both sides with respect to x, we get − sin ( x y ) { 1 d x d y } = y cos x sin x d x d y − sin ( x y ) − sin ( x y ) d x d y = y cos x sin x d x d y
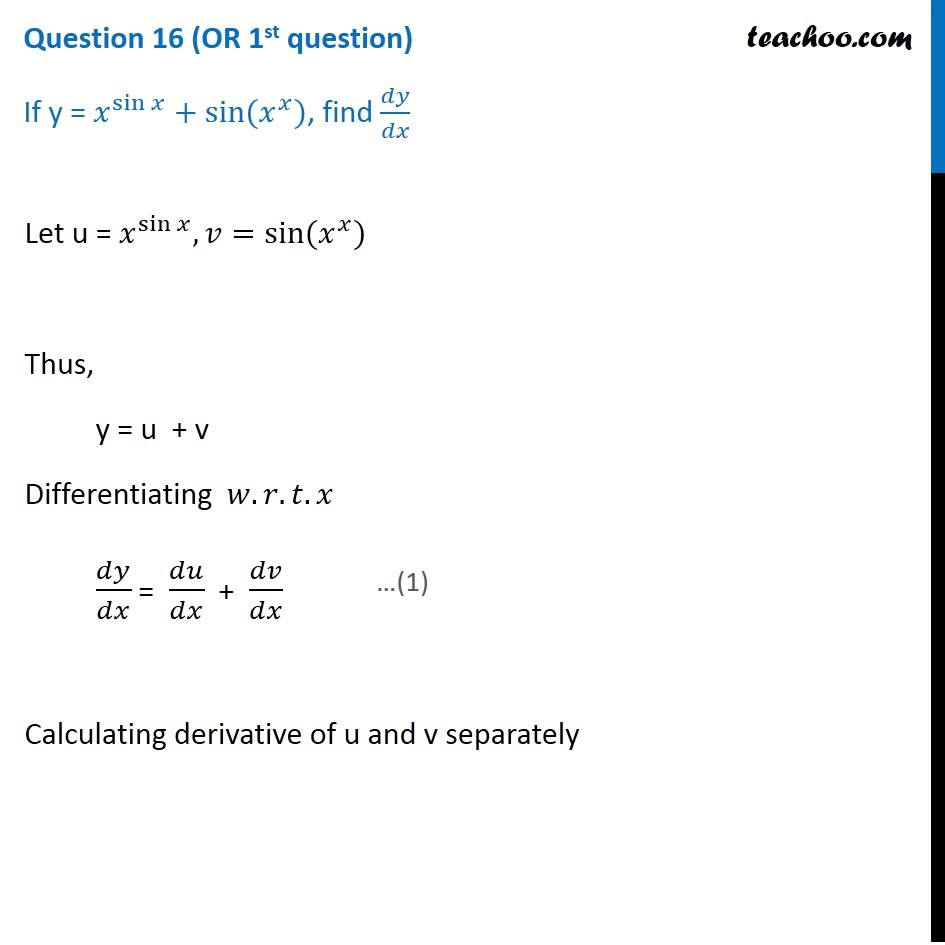



If Y X Sin X Sin X X Find Dy Dx Teachoo Cbse Class 12 Sam



Given That Y 1 Cosx Sinx How Do You Show That Dy Dx 1 1 Cosx Quora
Solution of dy/dx = (xlogx^2 x)/(siny ycosy) is (A) ysiny = x^2logx c asked in Differential equations by AmanYadav ( 556k points) differential equationsFind the general solution to sin(x) dy/dx cos(x) y = cos(6 x) y(x) = Find the unique solution that satisfies the initial condition y(?/2) = 4 y(x) = For this solution, d/ dx (?/2) = Hint Use the differential equation and the value of y(?/2) to find dy dt (?/2) dy/dx=(xtanx1secx)secxcos^2y We will use the Usual & the Rule of Implicit Differentiation xcosy=sin(xy)=sinxcosycosxsiny, (xsinx)cosy=cosxsiny Dividing by cosx*cosy, we get, (xsinx)/cosx=siny/cosy x/cosxsinx/cosx=siny/cosy, ie, tany=xsecxtanx d/dx(tany)=d/dx(xsecxtanx) sec^2y*dy/dx=x(secxtanx)1*secxsec^2x, =(xtanx1secx)secx
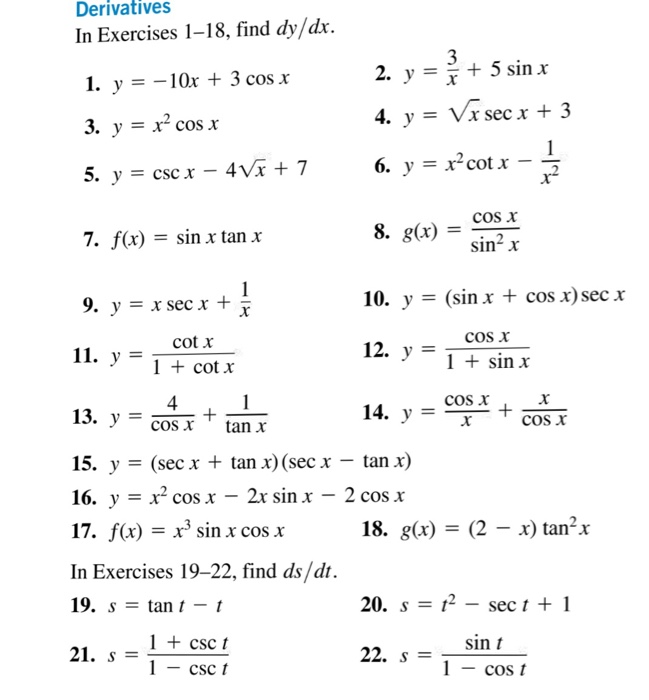



Derivatives In Exercises 1 18 Find Dy Dx 1 Y 10x Chegg Com




Find Dy Dx If Y Sin X Cos X Cos X Sin X Maths Continuity And Differentiability Meritnation Com
If it is y= (sinx)^0 then dy/dx= 0 coz then y=1 and if it is y=sin (x^0) even then answer would be one because the y=sin1 now sin 1 here is a constant and differentiation of constants is 0 so any ways y=sinx^0 its dy/dx =0 Hope this helpsFind dy/dx by implicit differentiation x sin(y) y sin(x) = 2 x cos(y) sin(x) y' x sin(y) y cos(x) Need Help?Find dy/dx when y=x^(cos x) (sin x)^ tan x Let y1=x cosx taking log on boths ides, we get logy1=cosxlogx now diffwrto x , we get (1/y1)dy1/dx=cosx(1/x)




If Y X Cosx Cosx Sinx Find Dy Dx
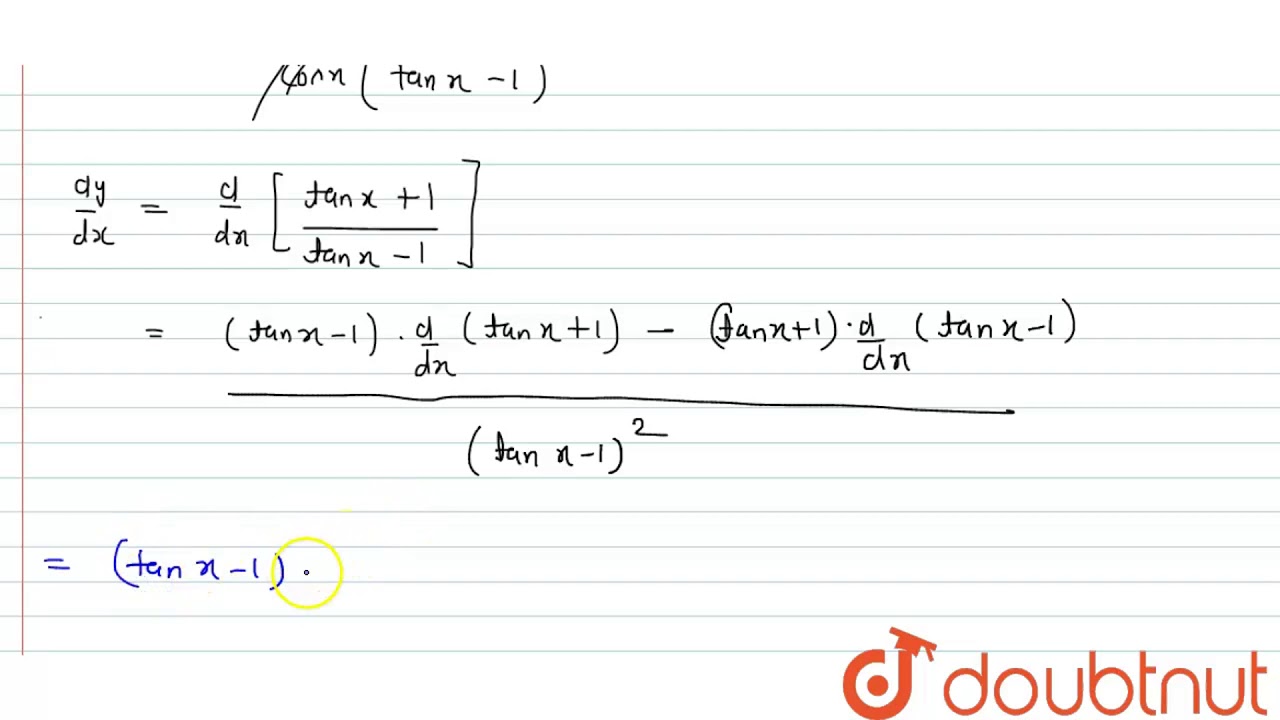



If Y Sinx Cosx Sinx Cosx Then Dy Dx At X 0 Is Equal To Youtube
Find dy/dx if y = log(sin x) Get the answer to this question and access a vast question bank that is tailored for studentsY = sin x cos x 5 dy/dx = cos x sin x d2y/dx^2 = sin x cos x Further, the maximum occurs at cos x sin x or cos x = sin x or x = 45⁰ The maximum value of y = sin 45 cos 45 5 = Solving for the derivatives brought upon us by the Product Rule math (\sin x)\ln\sin x (\frac {1} {\sin x}\cdot\cos x)\cos x = \frac {1} {y}\cdot\frac {dy} {dx} /math As a quick reminder, the derivative of the natural log of anything is math\frac {d} {dx} ln f (x) = \frac {1} {f (x)}\cdot f' (x) /math




Solve Cos X Cos Y Dy Sin X Sin Y Dx 0 The Answer Give In The Book Is Sin Y C Cos X Brainly In
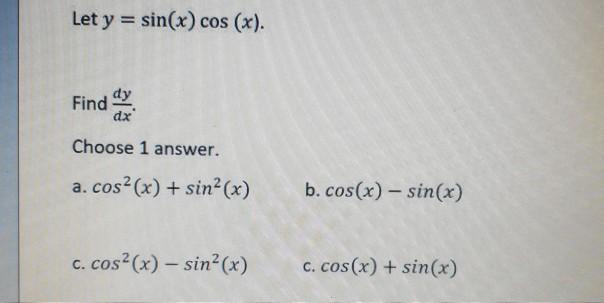



Let Y Sin X Cos X Find Dy Dx Choose 1 Answer A Chegg Com
$\cos(y)\frac{dy}{dx} \tan(x)\sin(y) = \sin(x)$ What would be a suitable substitution to get started?Here is a nice day again we are here to solve one of the problem Lets begin Given y = sin x cos x 5 Differentiating both sides we have => dy/dx = d/dx (sin x) d/dx (cos x) d/dx (5) we use rules d/dx (sin x) = cos x; Example 25 Find 𝑑𝑦/𝑑𝑥 , if y sin y = cos𝑥 y sin y = cos x Differentiating both sides by x 𝑑𝑦/𝑑𝑥 (𝑑(sin〖𝑦)〗)/𝑑𝑥 = (𝒅(𝐜𝐨𝐬〖𝒙)〗)/𝒅𝒙 𝑑𝑦/𝑑𝑥 (𝑑(〖sin 〗〖𝑦)〗)/𝑑𝑥 = − sin x 𝑑𝑦/𝑑𝑥 (𝒅(𝐬𝐢𝐧〖𝒚)〗)/𝒅𝒚 𝑑𝑦/𝑑𝑥 = − sin x 𝑑𝑦/𝑑𝑥



If Y X Sin X Cos X X 2 1 X 2 1 Find Dy Dx Sarthaks Econnect Largest Online Education Community



How To Sequence 1 2 Y Sin X Dy Cos X Dx 0 Quora
carlosego dy / dx = sin x / cos y We rewrite the equation (cos (y) * dy) = (sin (x) * dx) We integrate both sides of the equation sin (y) = cos (x) C We use the initial condition to find the constant C sin (3pi / 2) = cos (0) C 1 = 1 CX(sin y)dy/dx cosy y(sin x) cos x dy/dx = 0xsinydy/dx cos y y sin xcos xdy/dx=0 dy/dx (cos x x sin y)=y sin x cos y So dy/dx = (y sin x cos y)/(cosx x sin y)Find dy/dx y= (cos (x))/ (1sin (x)) y = cos (x) 1 sin(x) y = cos ( x) 1 sin ( x) Differentiate both sides of the equation d dx (y) = d dx ( cos(x) 1sin(x)) d d x ( y) = d d x ( cos ( x) 1 sin ( x)) The derivative of y y with respect to x x is y' y ′ y' y ′ Differentiate the right side of the equation



3
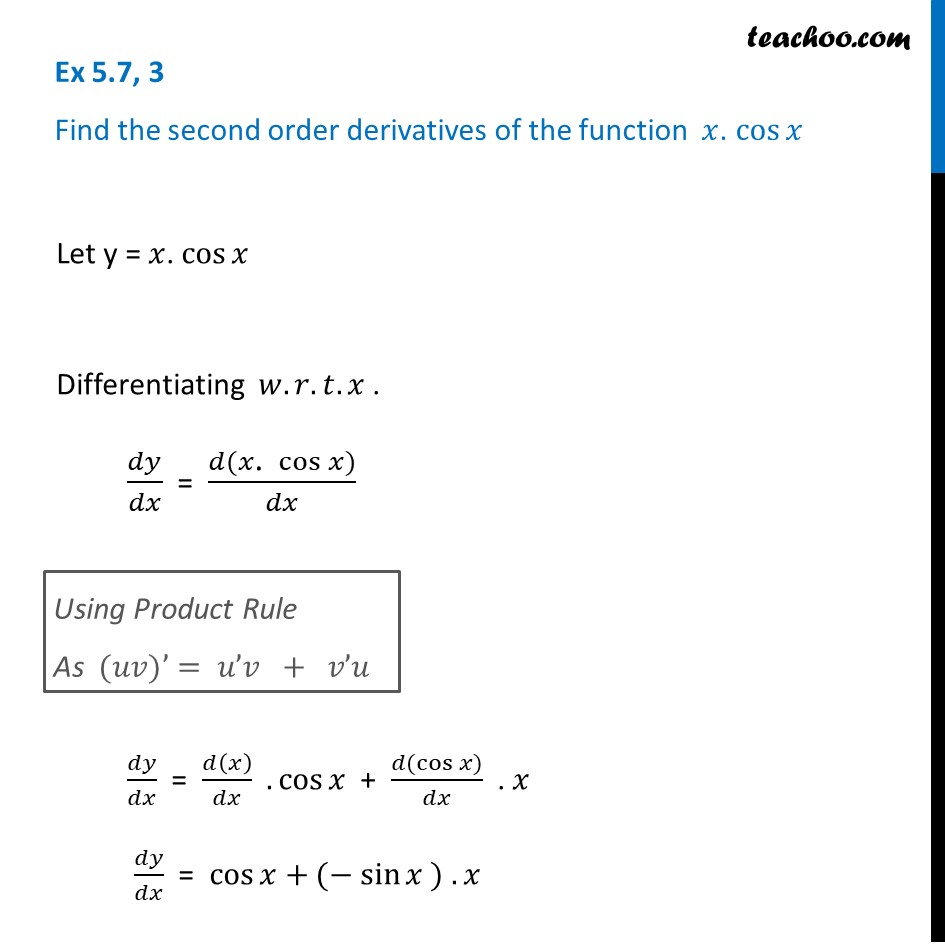



Ex 5 7 3 Find Second Order Derivatives Of X Cosx Ex 5 7
D/dx (cos x) = sin x; Let u = sinx, ∴ du dx = cosx Then, y = lnu, dy du = 1 u Combining, we get dy dx = 1 u ⋅ cosx = cosx u Substituting back u = sinx, we get = cosx sinx Notice how it equals to = ( sinxFind dy/dx y=(\sin x\cos x)^{2} 🚨 Hurry, space in our FREE summer bootcamps is running out 🚨




Find Dy Dx For Y X Tanx Sinx Cosx
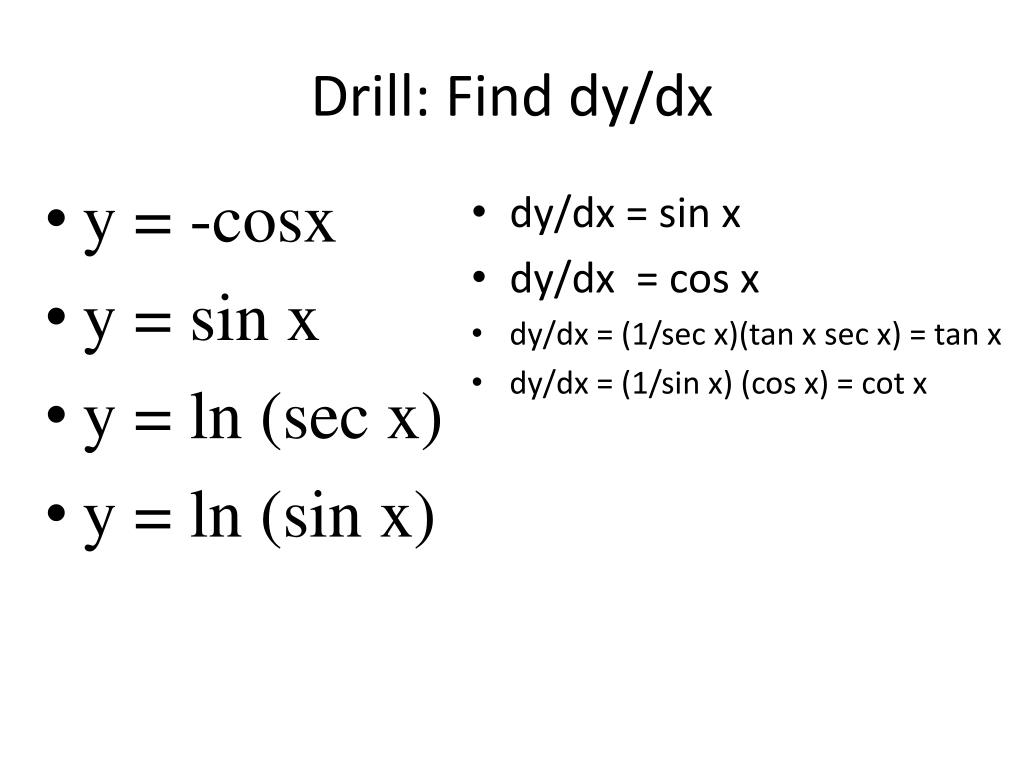



Ppt Drill Find Dy Dx Powerpoint Presentation Free Download Id
Ex 53, 8 Find 𝑑𝑦/𝑑𝑥 in, sin2 𝑥 cos2 𝑦 = 1 sin2 𝑥 cos2 𝑦 = 1 Differentiating both sides 𝑤𝑟𝑡𝑥 (𝑑 (sin2 𝑥 cos2 𝑦))/𝑑𝑥 = (𝑑 (1))/𝑑𝑥 (𝑑 (sin2 𝑥))/𝑑𝑥 (𝑑 (cos2 𝑦))/𝑑𝑥 = 0 Calculating Derivative of sin2 𝑥 & cos^2 𝑦 sepretaly Finding Derivative of 𝒔𝒊𝒏𝟐 𝒙 (𝑑 (sin2Find dy/dx y*sin (2x) = x*cos (2y) Get an answer for '`x sin(y) y sin(x) = 1` Find `(dy/dx)` by implicit differentiation' and find homework help for other Math questions at eNotes




Find Dy Dx If Y Cos X X Sin X 1 X Brainly In




Consider The Following Differential Equation Sin Y Chegg Com
Cos x dy/dx ysinx = 1 Divide by cosx both side dy/dx ysinx/cosx = 1/cosx ( sinx/cosx=tanx, 1/cosx =secx) WHICH GIVES dy/dx ytanx = secx now IF = e^integration tanx IF = e^lncosx = secx Solve \dfrac{dy}{dx}=\cos(xy)\sin(xy)Y = cos x / (1 sin x), find dy/dx derivative Watch later Share Copy link Info Shopping Tap to unmute If playback doesn't begin shortly, try restarting your device Up Next Let y = ex sin x^3 (tan x)x, find dy/dx Welcome to Sarthaks eConnect A unique platform where students can interact with teachers/experts/students to get solutions to
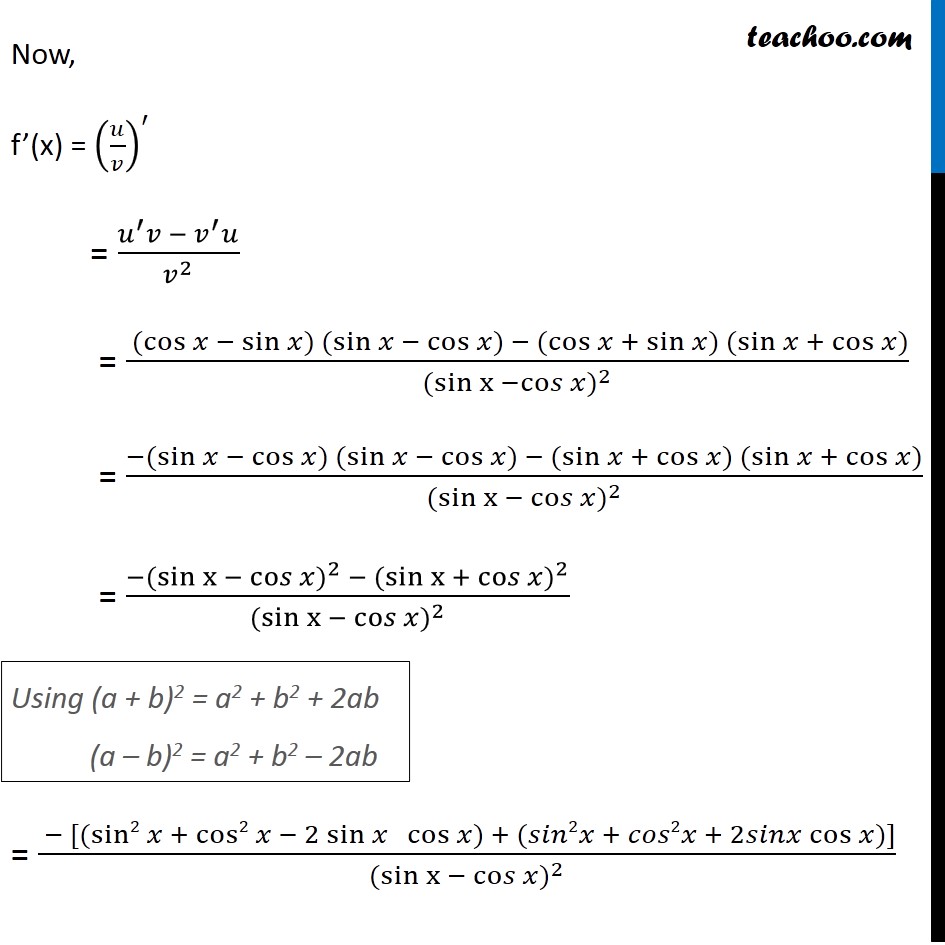



Misc 17 Find Derivative Sin X Cos X Sin X Cos X




If Y Sinx Cosx Cosx Sinx Prove That Dy Dx Sinx
Solve the differential equation $\frac{\mathrm{d}y}{\mathrm{d}x} = \cos(xy) \sin(xy)$ 2 Find the general solution of the differential equation $\frac{dy}{dx}\tan x\tan y=\cos x\sec y$ View Full Answer Mridula D, added an answer, on 1/7/14 Mridula D answered this IF ysiny = cos x differentiating on both sides, dy/dx cos y (dy / dx) = sin x taking dy/dx as common on LHS dy/dx ( 1cos y ) = sin x dy/dx = sin x / (1cos y)Stack Exchange Network Stack Exchange network consists of 177 Q&A communities including Stack Overflow , the largest, most trusted online community for developers to learn, share their knowledge, and build their careers
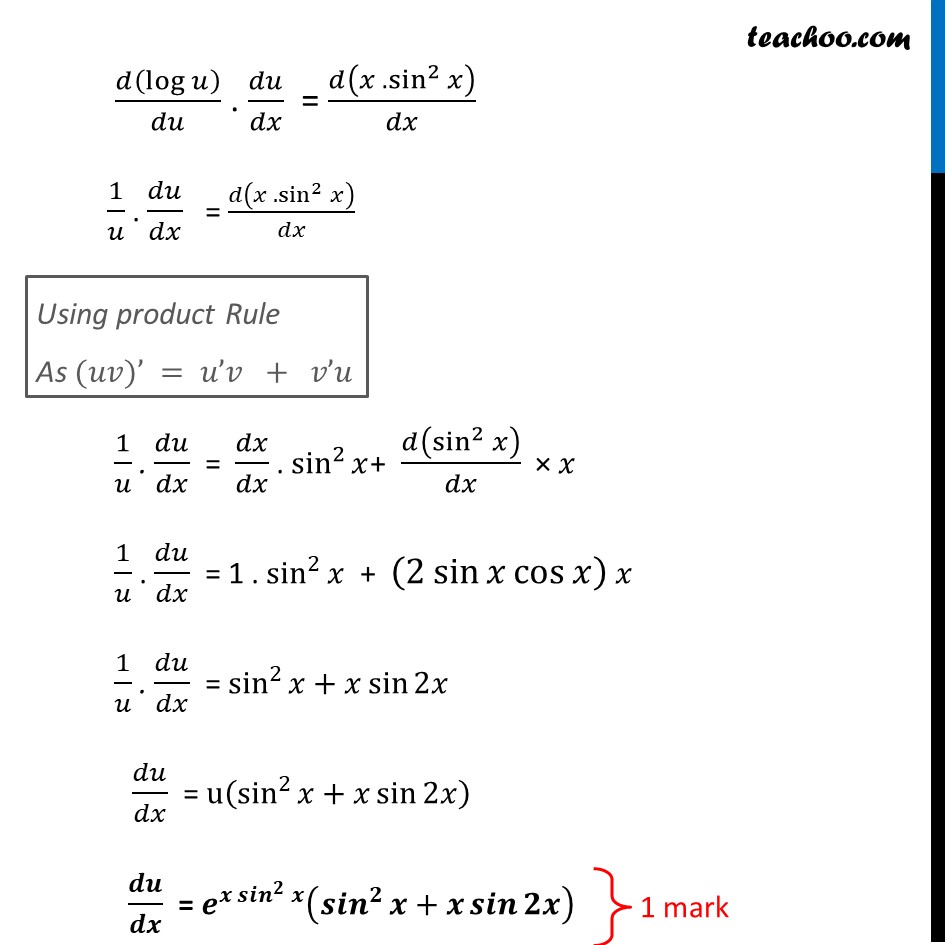



If Y E X Sin 2 X Sin X X Find Dy Dx With Video Teachoo




Find Dy Dx When If Y Cos X Sinx Cos X Sinx
যদি y = sqrt (sinx sqrt (sin x sqrt sin x )) তারপরে প্রমাণ করুন (dy) / (dx) = (cos x) / (2y1) x cosy = y cosx Taking log on both the sides, cos y log x= cos x logy cos y (1/x) log x (sin y) (dy/dx) = cos x (1/y)(dy/dx) logy (sinx) dy/dx ((siny log xDifferentiate {eq}y = \dfrac{5 \sin x}{x \cos x} {/eq} Application of derivatives This problem involves using the Quotient rule of differentiation to find the derivative of a given function



Q Tbn And9gcrnhrbok2vv Trw3qxe7u2d6je2zt9wdoj F7iigkox2bbxi17l Usqp Cau




What Is The Derivative Of Sinx Cosx Using First Principle Quora
If Y X 3 5 And X Changes From 3 To 2 99 Then The Approximate Change In Y Is If y 2 = ax 2 bx c, where a, b and c are constant, then y 3 d 2 y/dx 2 is If you spill a chemical toilet cleaning liquid on your hand, your first aid would be If z = tan (y ax) √(yax), then z xxa 2 z yy is equal to If Z 1 Iroot 3 By Root 3 I Then Bar ZPLEASE SOLVE " dy/dx=sin(xy)cos(xy) " and please tell me the genaral solution of the first degree differential equation of given Put xy = v 1dy/dx = dv/dxShow \iint \cos\frac {xy} {xy}\, dx\,dy=\frac {\sin1} {2} over the region R Let R be the region bounded by x y = 1,x = 0,y = 0 Show ∬ cos xyx−y Your way for solving the problem is right but you may lose the proper range of u and v Try to draw the triangle region and then find the corresponding vertices according to the




Y Sin X3 5 Find Dy Dx Dy Dx Type Sin X For Chegg Com
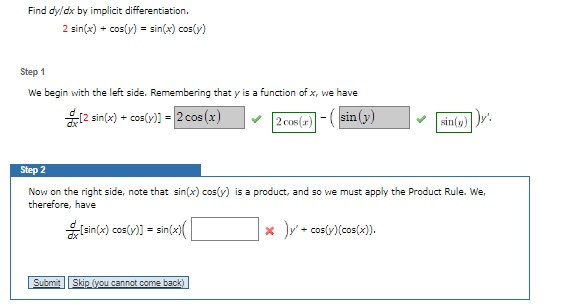



Find Dyldx By Implicit Differentiation 2 Sin X Chegg Com
D/dx (constant term) = 0 => dy/dx = cos x ( sin xY = e x sin x On differentiating, we get d x d y = sin x d x d e x e x d x d sin x d x d y = sin x e x e x cos x ∴ d x d y = e x (sin x cos x)
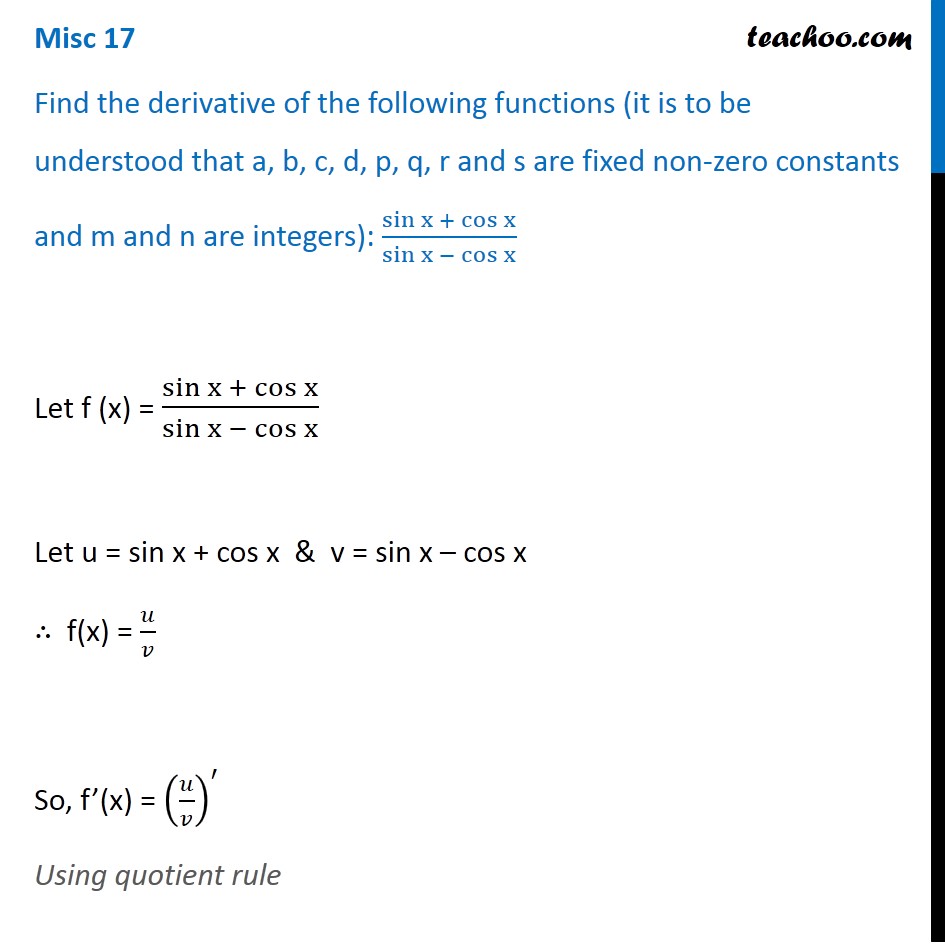



Misc 17 Find Derivative Sin X Cos X Sin X Cos X



What Is The Area Bounded By Curve Y Sinx Y Cosx X 0 And X Pi 2 Quora




If Y Sin X 9 Cosx Then Dy Dx At X 0 Is Equal To




Find Dy Dx By Implicit Differentiation X Sin Y Y Sin X 8 Wyzant Ask An Expert



1




Find Dy Dx When Y Is Equal To Sin X Cos X Maths Continuity And Differentiability Meritnation Com




Y Sin X Cos X Find Dy Dx Brainly In



Find Dy Dx If Y Cosx X Sinx 1 X Sarthaks Econnect Largest Online Education Community




The Solution Of Dydx Ycosx Siny Ysinx Xcosy X 0 Is
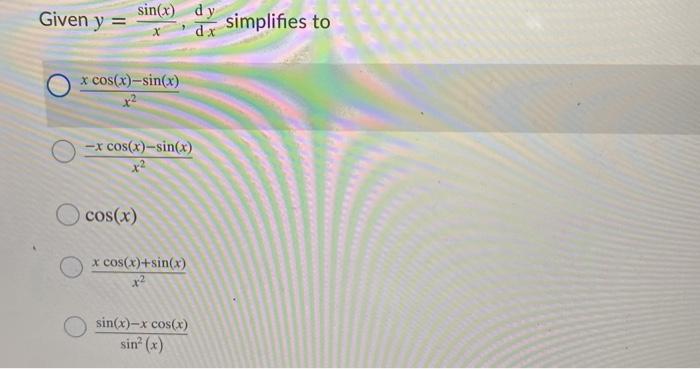



Solved Given Y Sin X Dy Dx Simplifies To Cos X Sin Chegg Com
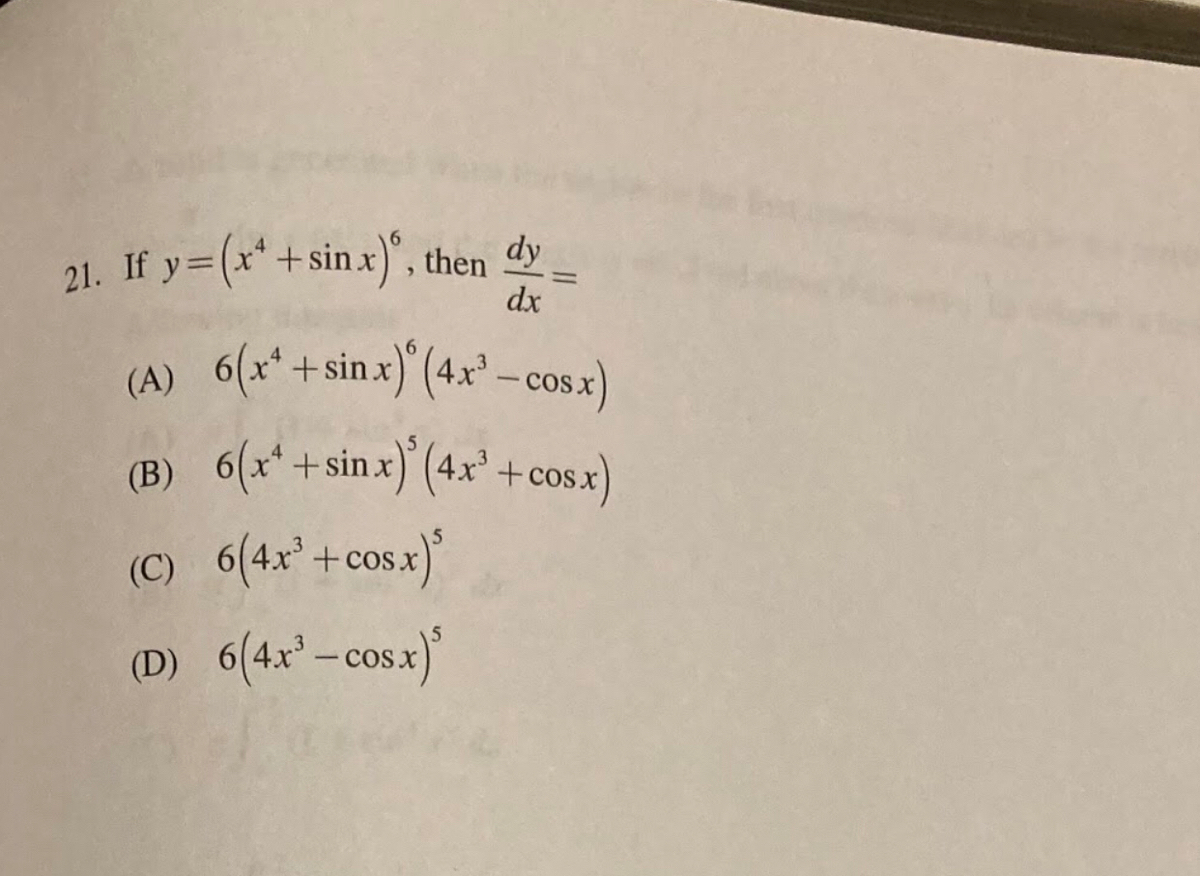



Answered 21 If Y X Sin X Then Dy Dx A Bartleby




Evaluate Oint C Y Sin X Dx Cos X Dy Mathematics Stack Exchange




Given X Cos Y Y Cos X 1 Find Dy Dx By Implicit Chegg Com




If Y Cosx Sinx Then Dy Dx At X 2pi 3 Is




If Y Sin X X Cos X Find Dy Dx



What Is Math Frac Dy Dx Math If Math X Cos Y Y Cos X 1 Math Quora



What Is The Derivative Of Sin X Cos X Quora



Sinx Dy Dx Cos X Y Cos X Sin 2 X Youtube




If Y Tan 1 Sinx Cosx Cosx Sinx Then Find Dy Dx Brainly In



Solve Cos X Dy Y Sin X Y Dx Mathematics Stack Exchange




If Y Sqrt X Sin X Cos X Find Dy Dx Youtube




If Y Sinx Cosx Sinx Cosx Then Find Dy Dx Of X 0 Maths Limits And Derivatives Meritnation Com




Find Dy Dx If Y Cosx X Sinx 1 X Youtube



Solve The Differential Equation Sin X Dy Dx Cos Chegg Com
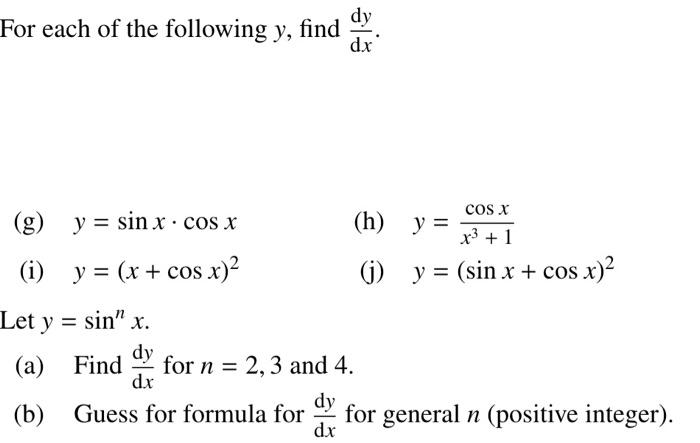



For Each Of The Following Y Find Dy Dx Cos X Y Sin Chegg Com



Derivatives Of Sin X And Cos X Video Khan Academy




Question 13 On Given Cos X Given Cos X Y Sin X Chegg Com



If Y Sin X X Cos X Find Dy Dx Youtube




Find Dy Dx If Y Tan 1 Cos X Sin X Cos X Sin X Maths Continuity And Differentiability Meritnation Com
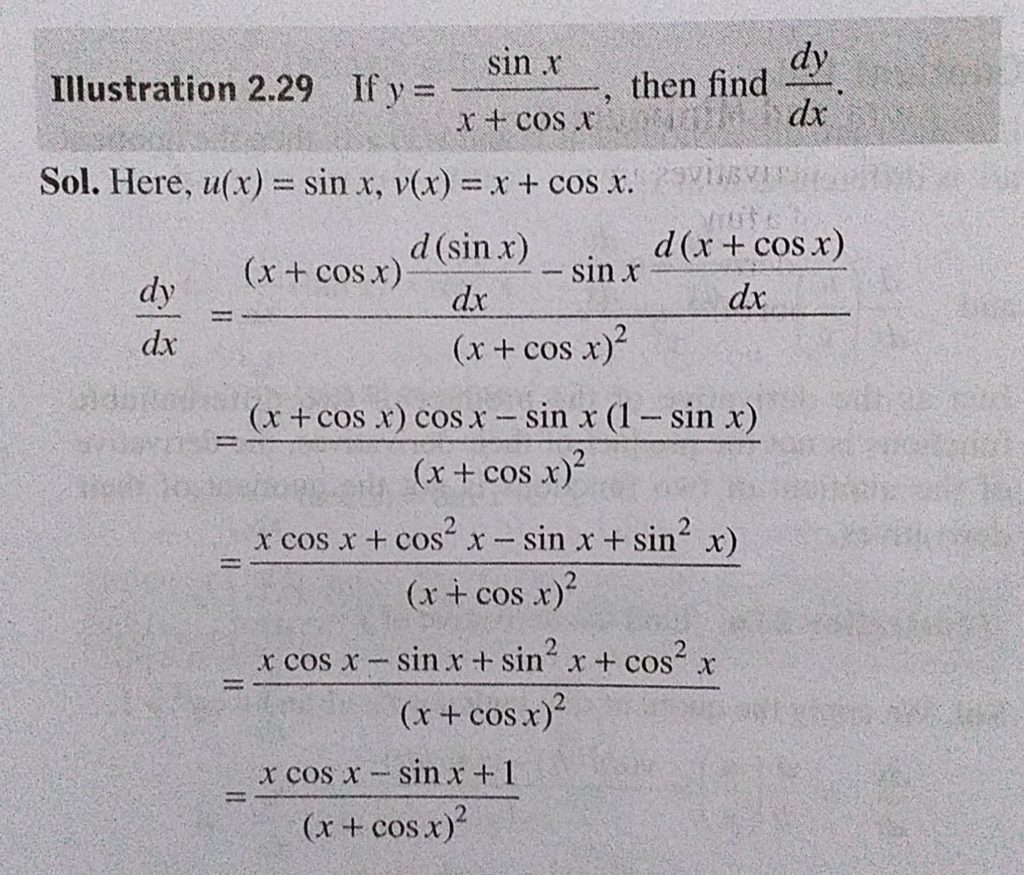



If Y Sinx X Cosx Then Find Dy Dx Sahay Lms
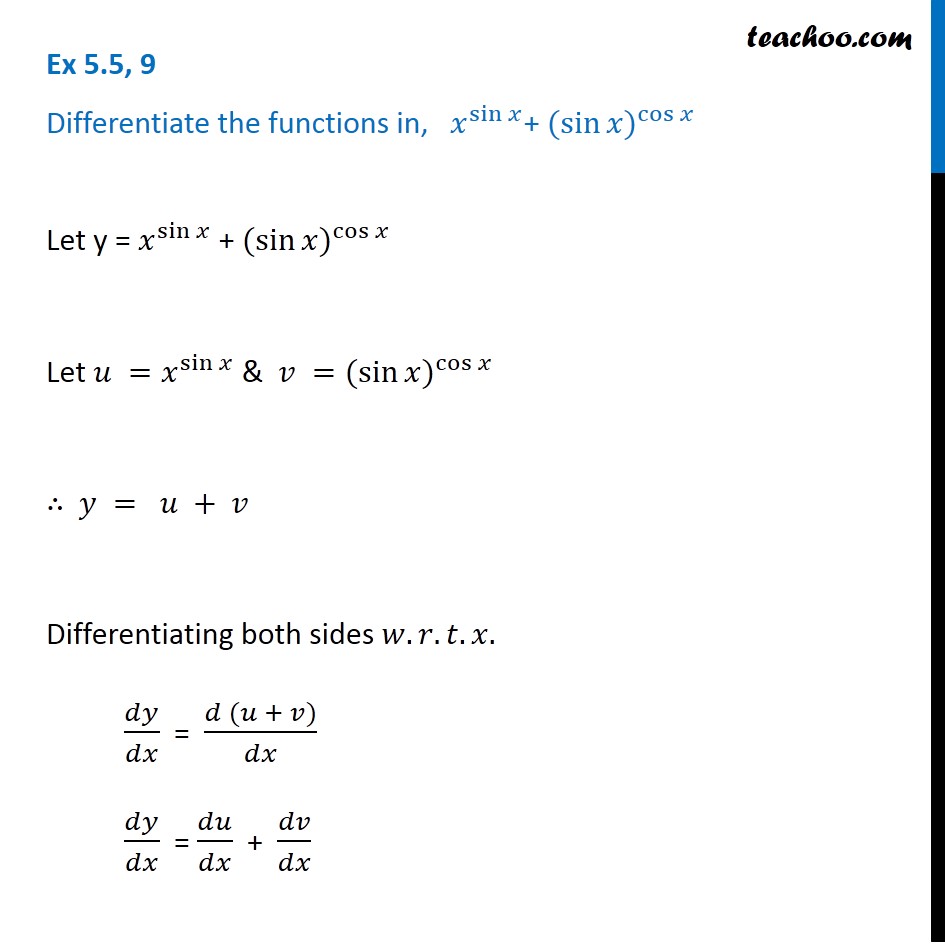



Ex 5 5 9 Differentiate X Sin X Sin X Cos X Chapter 5 Class 12




If Y Cosx Sinx Cosx Sinx Prove That Dydx Y 2 1 0




Cos X Dy Dx Y Sin X 1 Y Pi 4 0 Solve By Chegg Com




Ex 5 5 9 Differentiate X Sin X Sin X Cos X Chapter 5 Class 12




If Y Sinx Tanx Cos X Secx Find Dy Dx Youtube




If Y Sinx Cosx Sinx Cosx Then Dy Dx At X 0 Is Equal T
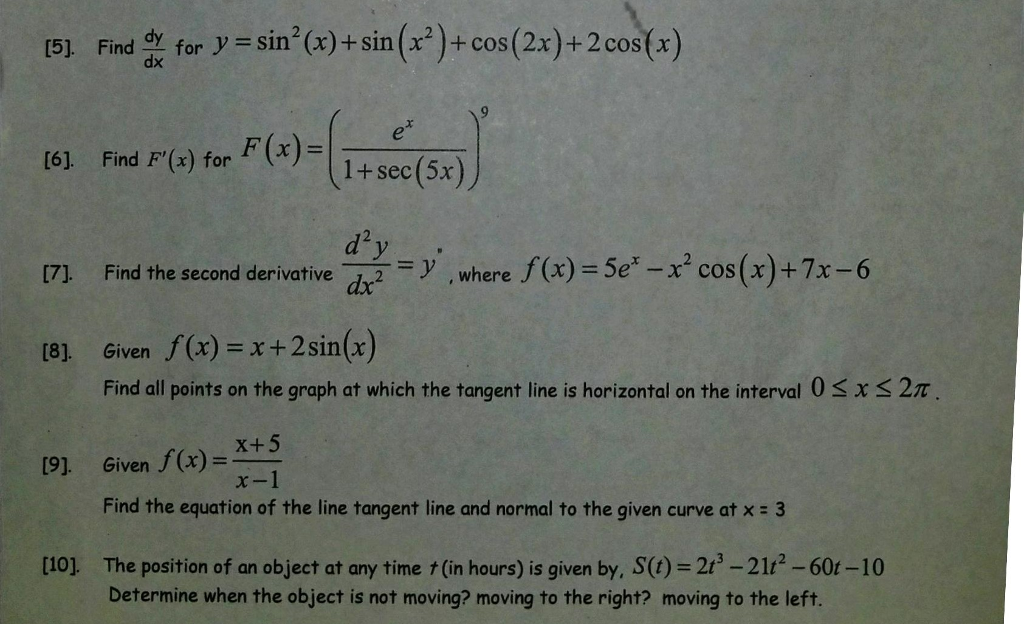



Find Dy Dx For Y Sin 2 X Sin X 2 Cos 2x Chegg Com



What Is The Value Of Dy Dx If X Cos Y Sin X Y Quora



How To Find The Dy Dx Of Siny Cosx Cosy Sinx Quora




3 12 Find Dy Dx By Implicit Differentiation 9 4 Cos Chegg Com




Differentiate Y Sinx Cosx Youtube



1




If F X Cos X Sin X Then Dy Dx At X 2pi 3 Is Eq
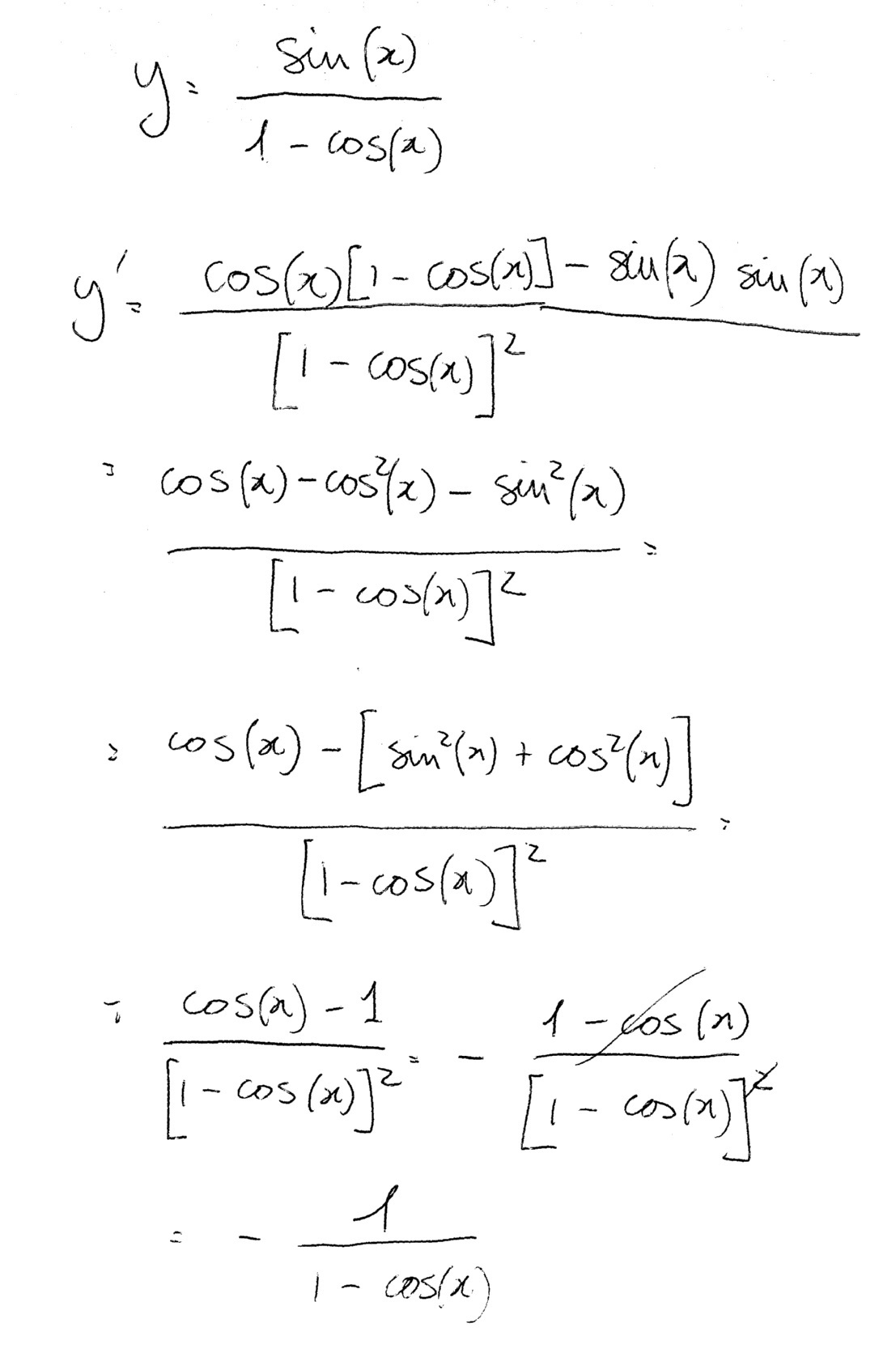



What Is The Derivative Of Sin X 1 Cos X Socratic
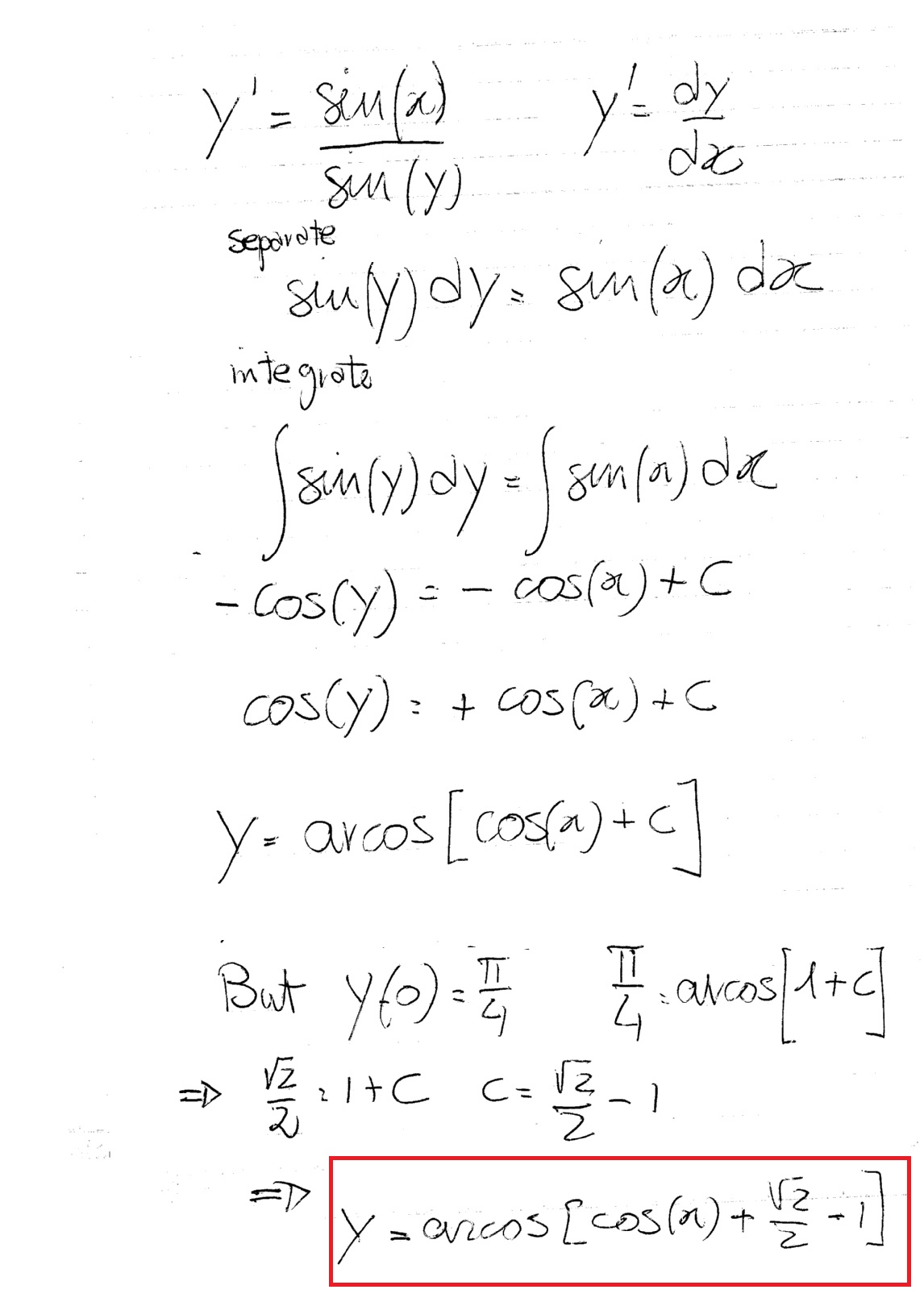



How Do You Solve The Initial Value Problem Y Sinx Siny Where Y 0 P 4 Socratic
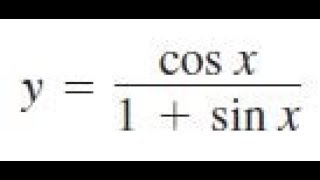



Y Cos X 1 Sin X Find Dy Dx Derivative Youtube
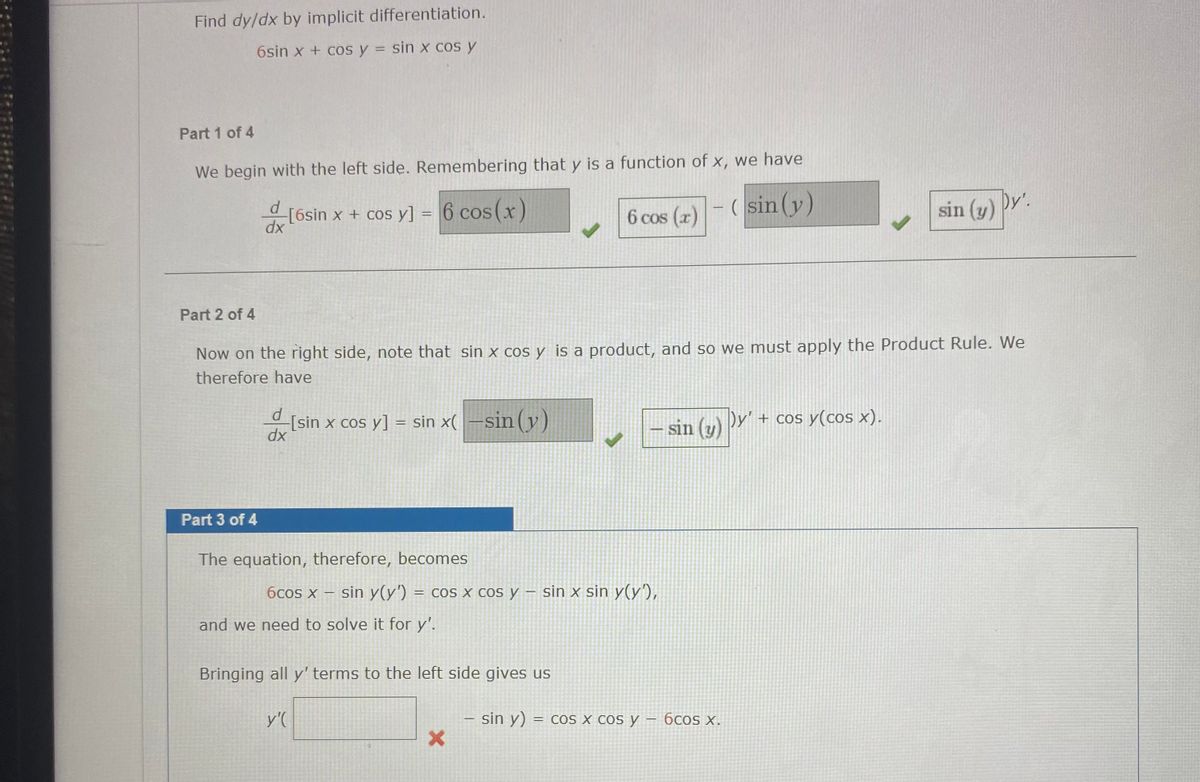



Answered Find Dy Dx By Implicit Differentiation Bartleby
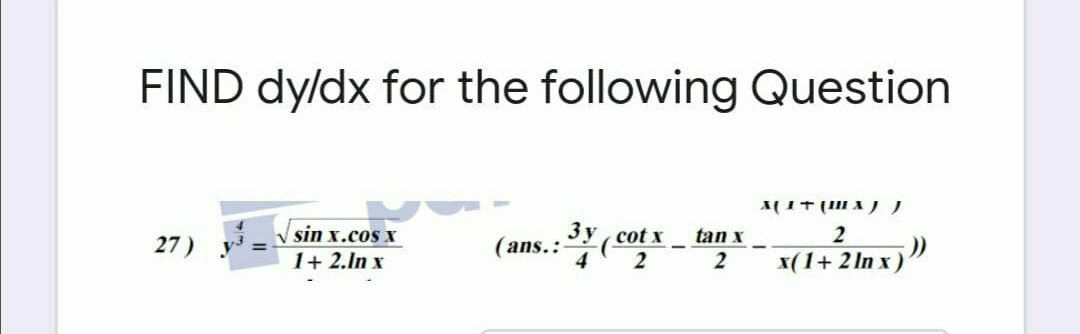



Answered Find Dy Dx For The Following Question Bartleby




Derivatives Of Sin X Cos X Tan X Eˣ Ln X Video Khan Academy
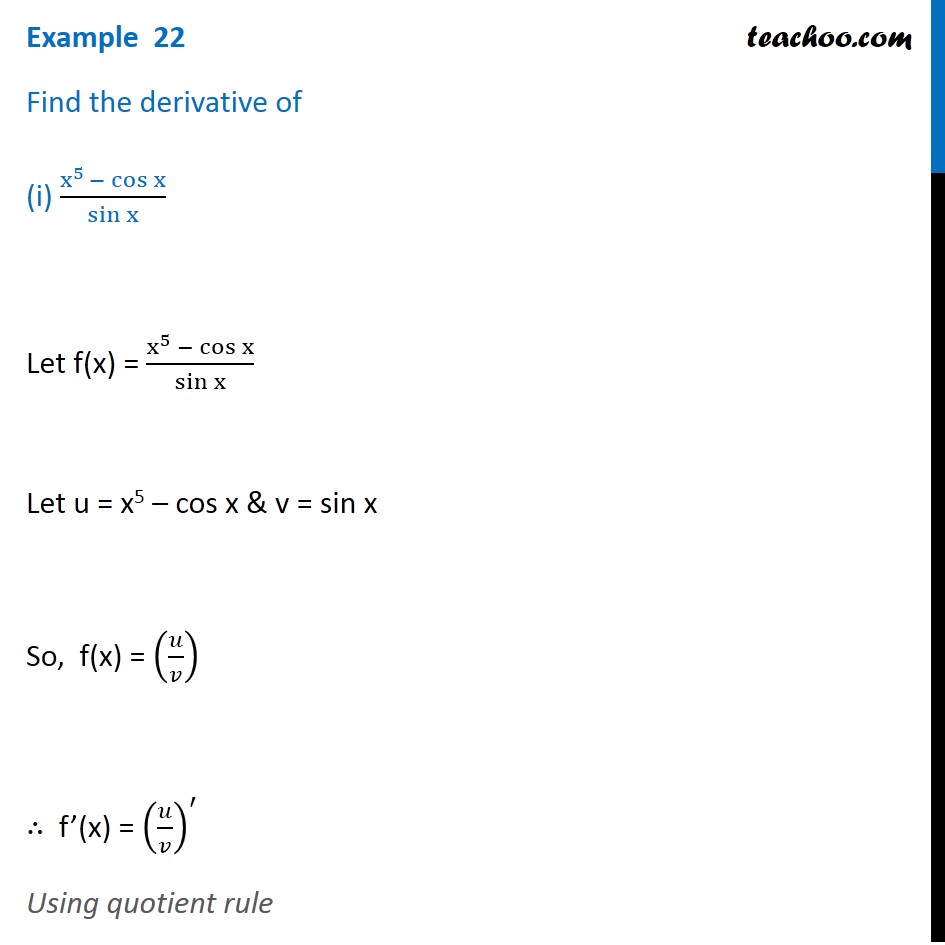



Example 22 Find Derivative Of I X5 Cos X Sin X Examples
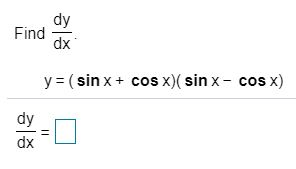



Solved Dy Find Dx Y Sin X Cos X Sin X Cos X Dy Dx 11 Chegg Com
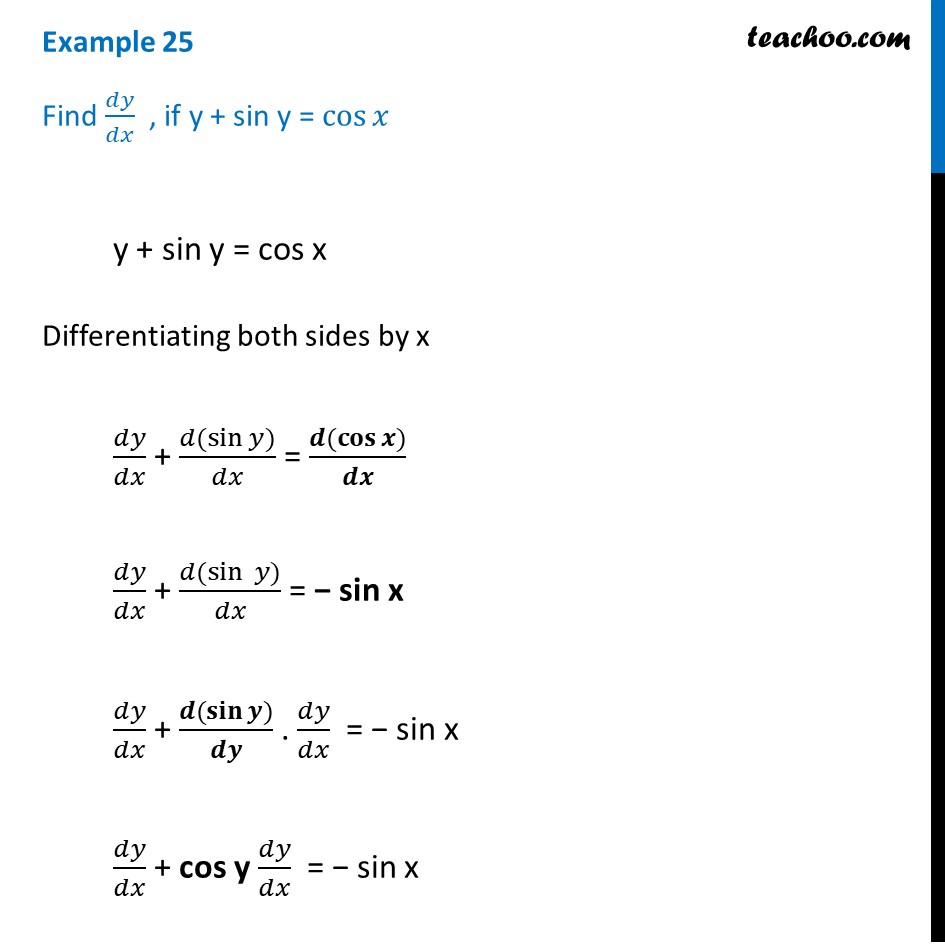



Example 25 Find Dy Dx If Y Sin Y Cos X Chapter 5 Class 12




Y Sin X Cos X Sec X Find Dy Dx Derivative Youtube




If Y Sin Cosx 2 Find Dy Dx




Linear Differential Equation Cos X Dy Dx Sin X Y 1 Youtube




If Y Sin X Cos X Then Dy Dx Brainly In




Y Sinx Cosx Sinx Cosx Find Dy Dx For The Given Function Y Wherever Defined Brainly In
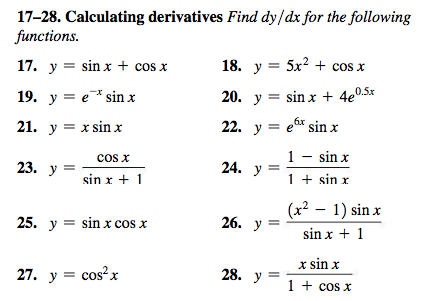



17 28 Calculating Derivatives Find Dy Dx For The Chegg Com
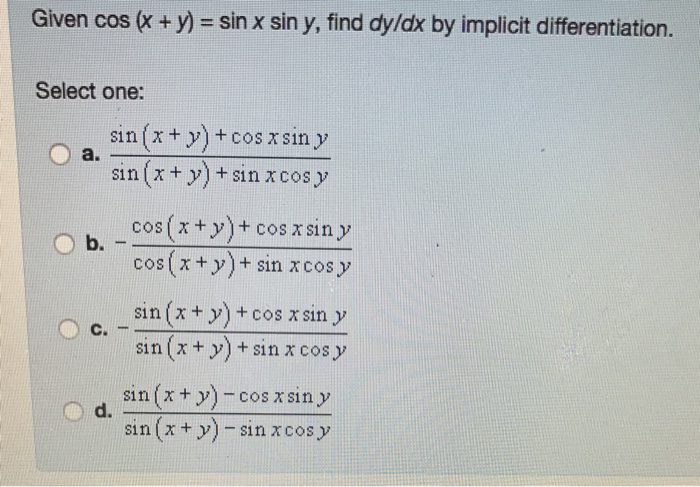



Given Cos Sin X Sin Y Find Dy Dx By Implicit Chegg Com




If Y Sin X X Cos X Then Find Dy Dx




If X A Cosx Xsinx Y A Sinx Xcosx Then Find D Y Maths Meritnation Com
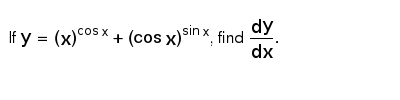



If Y X Cosx Cosx Sinx Find Dy Dx



If Cos X Y Y Sin X Find Dy Dx Youtube




If Y Sin X 2 Cos X 2 2 Then Find Dy Dx At X P 6 Brainly In
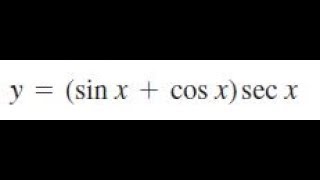



Y Sin X Cos X Sec X Find Dy Dx Derivative Youtube
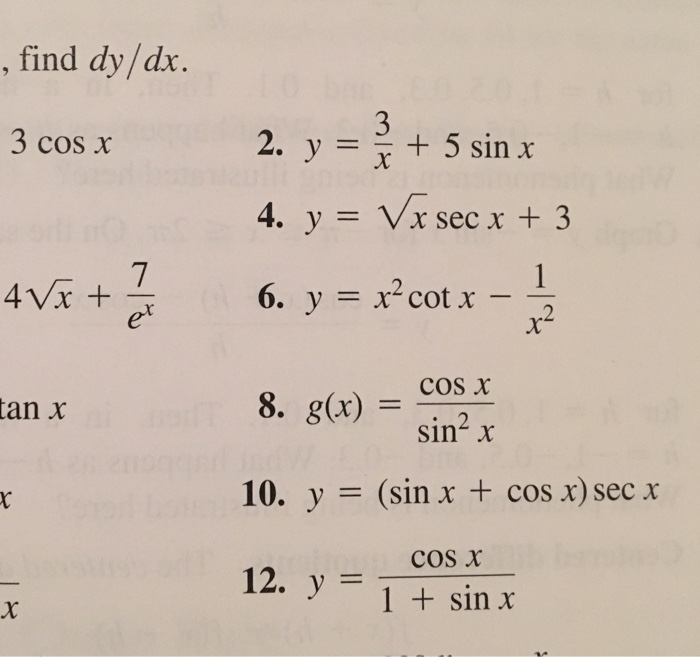



Find Dy Dx 3 Cos X 4vx Cos X 8 G X 10 12 Y Lt Chegg Com
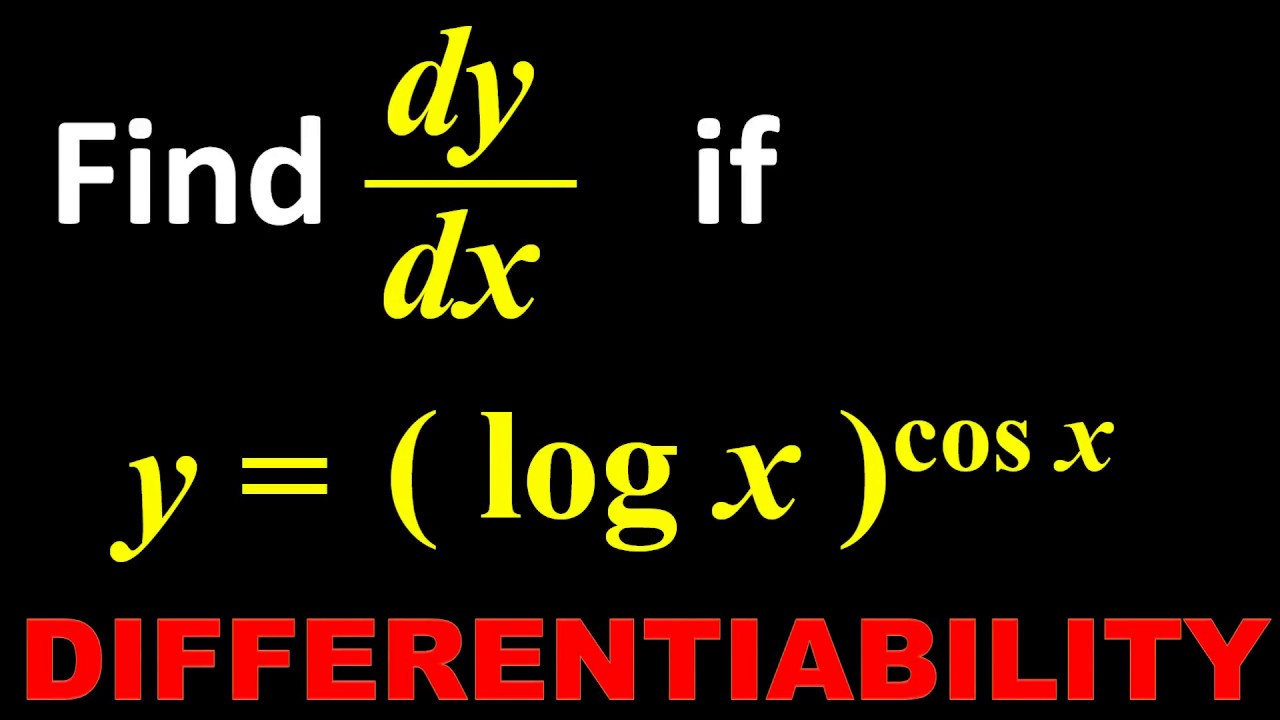



23 Derivative Find Dy By Dx Of Y Log X Cos X Youtube




Pin En Differential Equations Videos



If Y Sin X 0 Then Find Dy Dx Quora



If Sin X Cos X Sin X Cos X Then Dy Dx At X 0 Is Sarthaks Econnect Largest Online Education Community




If Y Sinx Tanx Cos X Secx Find Dy Dx




Solve The Differential Equation X Dy Dx Y X Cosx Sinx Given That Y Pie 2 1 Maths Differential Equations Meritnation Com



If Cosx Y Siny X Find Dy Dx Sarthaks Econnect Largest Online Education Community
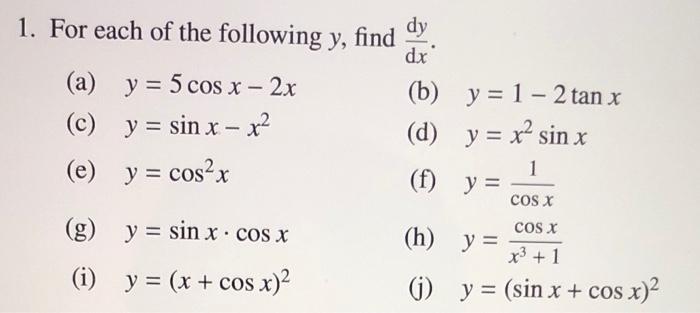



1 For Each Of The Following Y Find Dy Dx A Y 5 Chegg Com
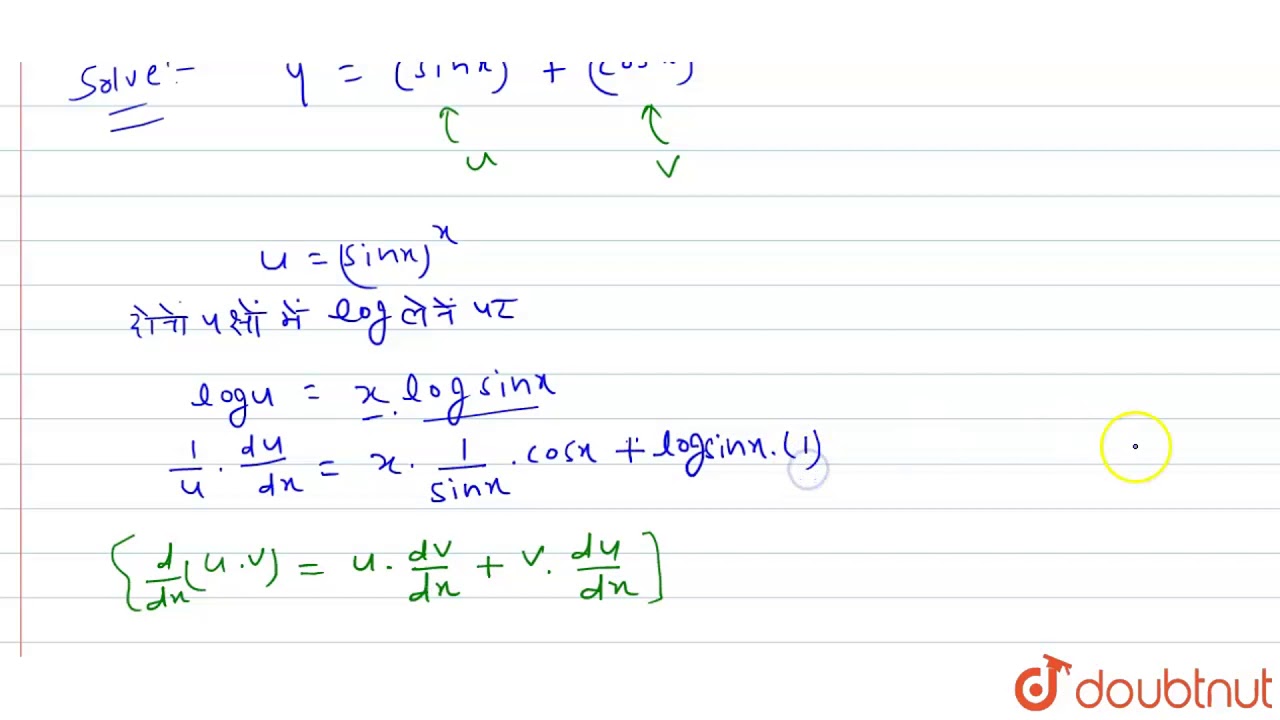



यद If Y Sinx X Cosx Tanx Find Dy Dx न क ल Youtube



How To Solve Sin X Dy Dx 3y Cos X Using An Integration Factor Quora




Find Derivative By First Principle F X Sin X Cos X Video




Find Dy Dx Of X Cos Y Sin X Y Socratic



0 件のコメント:
コメントを投稿